The Siphon or Syphon is the most widely used instrument in fluid mechanics. Let us consider, there are two tanks kept at different elevations and a barrier is there between them. How can the liquid be transferred from the higher elevation tank to the lower elevation tank? Here, the siphon plays an important role to transfer the liquid. Let’s explore!
What is Siphon or Syphon? Definition, Meaning
Basics & Meaning of Siphon
The siphon basically an inverted U-tube or pipe filled with water. The filling of the pipe or tube is simple and many ways it can be done. Siphon is an Ancient Greek word. It is written as ‘’σίφων’’. In Roman, it is written as ‘síphōn‘, which means “pipe or tube“. It is also spelled as Syphon. It is the same as the priming of pumps.
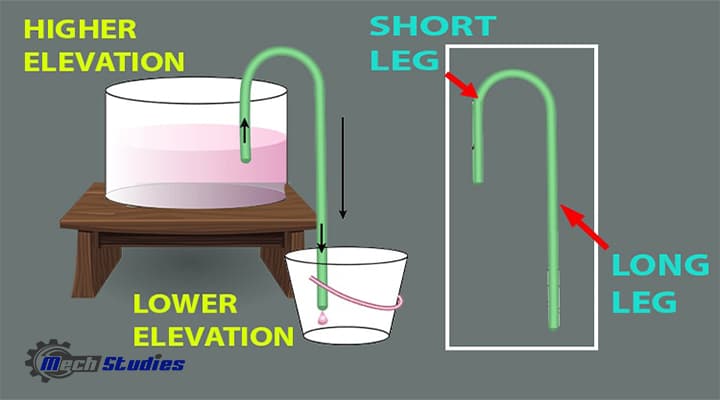
- Each siphon has two legs. One is long with respect to others,
- The short leg is connected to the tank kept at the higher elevation
- Another leg is connected to the tank kept at the lower elevation
- The action depends upon a few factors, like cohesive forces or pressure differences, etc.
Siphon or Syphon Definition
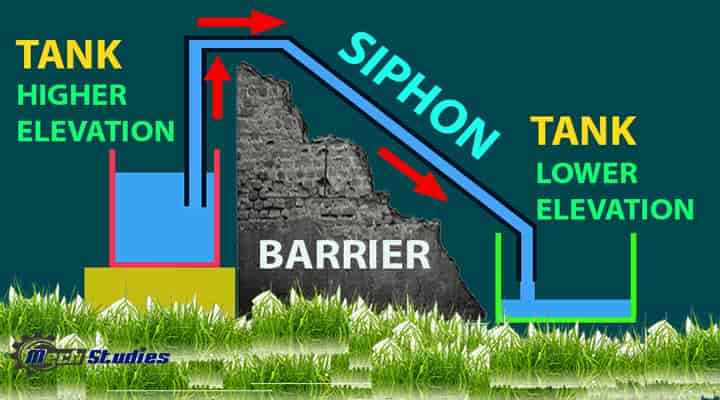
A syphon is an inverted u-shaped tube or pipe used to transfer liquid from a higher elevation tank to a lower elevation tank when both the tanks are separated by a high-level barrier.
Function of Siphon
The purposes of Syphon are, as follows:
- If two tanks are kept at different elevations and there is a barrier between them, the siphon is used to transfer liquid from the tank at a higher elevation to the tank at a lower elevation.
- The net result is that the liquid passes from the reservoir to a lower level with a continuous flow.
- No external energy is required for liquid transfer.
- The flow continues unless and until the water level reaches the output end of the siphon tube.
History of Syphon
Their earliest known use dates back to the Egyptians approx 1500 BC. Tesibius of Alexandria was invented a usable siphon.
Difference between Syphon & Leaky Bucket
How do you differentiate syphon and a leaky bucket? It’s very simple! The main difference between a syphon and a leaky bucket:
- Liquid transfer happens without any hole in the tanks whether a leaky bucket means a lot of holes.
- Liquid transferred to a higher level tank before draining out to a lower level tank.
How Does a Siphon or Syphon Work? Working Principle
When we discuss the working principle of the siphon, from the definition of siphon, there are a few things that come to our mind?
- Why the liquid level rises?
- Why does the liquid flow continuously?
Let’s try to analyze to understand the siphon working philosophy.
Step#1: Atmospheric pressure and vacuum
Let us take a tube of one end closed and another end is open. Now, fill the tube with a liquid and raised up the closed-end while the lower end is immersed in a liquid reservoir. If we observe the tube closely, we will see the liquid in the tube stays up to a certain height. But, if we increase the height of the tube further, the water level does not rise again. Why? It is because this height depends on:
- the density of the liquid and
- the pressure of the air outside the tube.
Air pressure is reduced with height or elevation. That is the reason; we face difficulty breathing at the top of the mountain.

Look at the below diagram,
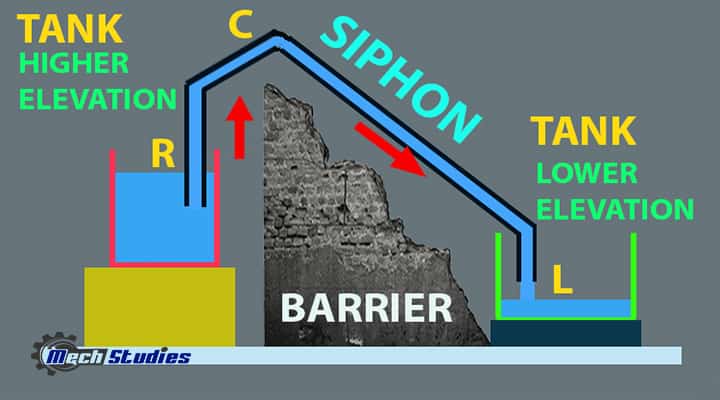
- C = Summit of the siphon, i.e., the highest point of the siphon
- R = Reservoir or tank kept at a higher elevation
- L = Tank kept at a lower elevation
- H = Height difference between the tank, H and summit, C
In this diagram, point C is at certain height from the tank surface H. It means the height is increased towards summit C; pressure will be decreased gradually. At point C, the pressure will be below atmospheric pressure, and water will rise in the shorter column and liquid flow will be started.
As per theoretical calculation, air sustains up to a liquid height of 10 meters (32 ft. of water), and up to that siphon will work. If the height of the tube is further increased, dissolved air and other gases will come out and form a vacuum in the summit and interrupt the continuity of liquid flow. As a result, the siphon will not work.
in the summit and interrupt the continuity of liquid flow. As a result, the siphon will not work.
Step#2: Working with cohesive forces
If we analyze, with water, we will see both the end of the siphon have the same pressure, that is atmospheric pressure. Do you think atmospheric pressure is the main factor to have siphon work? No, the siphon can even work in a vacuum condition also. However, this is applicable to very cohesive liquids only.
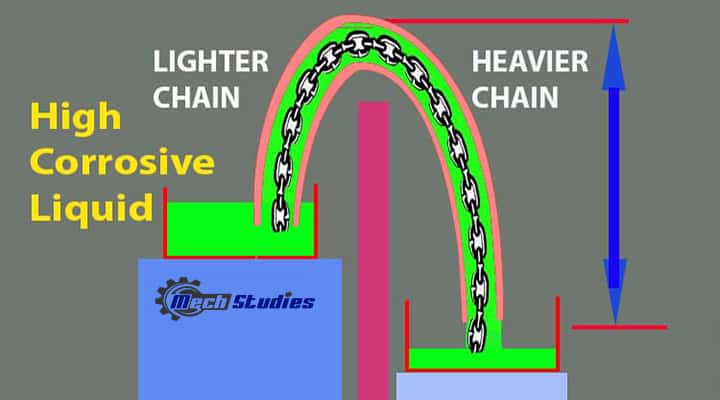
Molecular attraction in high cohesive liquids is very strong and they can maintain a chain-like action in the inverted tube and maintain a continuous flow.
Step#3: Gravitational Potential Difference
Do you think atmospheric pressure or weight difference of liquid sustains the flow within the siphon? No! It’s all about gravitational potential difference. There will be a gravitation potential difference between the liquid level in the tank and at the output siphon’s opening. This difference makes an unbalanced system and stores potential energy. As a result, the liquid flow will be continued. Let’s focus on two factors atmospheric pressures and tube heights. Now, the taller column is connected to the lower tank.
- A tall water column is on the lower level tank
- Normally gravity will pull the liquid down
- Liquid column down means there will be a reduction of pressure at the top of the siphon
- The reduction of the pressure will create an unbalance in the small column
- There will be a gravity force in the shorter column as well
- This gravity force is comparatively less with respect to the reduced pressure
- So, the shorter column will rise to the top of the siphon
Hence, the liquid starts to flow from a higher elevation tank to a lower elevation tank continuously.
Mathematical Expression of Siphon
Let’s consider,
- p: Pressure difference between two points,
- ρ: is the liquid density,
- g: gravitational acceleration
- v: water velocity at the bottom of the siphon
- z: elevation
- g: gravitational acceleration
Hence, we all know,
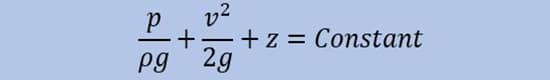
This expression is derived from, Bernoulli’s equation
Example of Siphon Calculation
Siphon Problem: Let’s consider a siphon, which is used to drain water from a tank, T1.
- Siphon diameter = 4 cm,
- The siphon outlet end is 2m below the surface in the tank, T1
- The peak point of the summit is 1.5 m above the water surface.
Calculate the discharge and pressure of the fluid at the discharge point. Neglect friction.
Solution: Given Data
Calculate discharge
Dia of tube, d=d1=d2=d3 = 4 cm = 0.04 m
Formula to use: Bernoulli’s equation for frictionless flow is
Discharge (Q) = cross-sectional area x velocity
Calculations
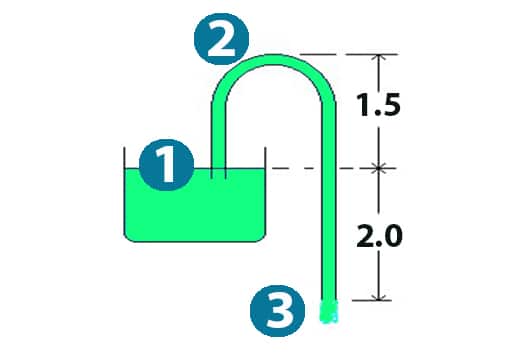
We will apply, Bernoulli’s equation at point 1 and 3,
At point, 1 (Energy level of the fluid in the tank surface)
- Pressure = p1 = 0 N/m2
- Elevation = z1 = 0 m
- Velocity = v1= 0 m/sec
At point, 3 (Energy level of the fluid in the discharge point)
- Pressure = p3 = 0 N/m2
- Elevation = z3 = -2 m
- Velocity = v3= ?
Therefore,
p/ρg + v2/2g + z = Constant
Hence, considering point 1 & 3, applying Bernoulli’s equation
- p1/ρg + v12/2g + z1 = p3/ρg + v32/2g + z3
- 0 + 0 + 0 = 0 + v32/2g + z3
- 0 = v32/2g + z3
- 0 = v32/2g +(-2)
- v32/2g = 2
- v32 =2 x 2g
- v32 =2 x 2 x 9.81
- v32 = 39.24
- v3 = 6.3 m/s
Discharge, Q
- Q = Area x Velocity
- Q = π d32/4 x v3 [as Area = π d32/4]
- Q = 3.14 x 0.042/4 x 6.3 [as d = 0.04]
- Q = 0.00791 m3/s
- Q = 28.5 m3/hr
Applying Bernoulli’s theory, at points 1 and 2,
- P2 = 0 N/m2(g)
- z2 = 1.5 m
- v2 = v3 = 6.3 m/sec (since, cross-sectional are same)
Let’s write the equation,
- p1/ρg + v12/2g + z1 = p2/ρg + v22/2g + z2
- 0 + 0 + 0 = p2/ρg + v22/2g + z2
- 0 + 0 + 0 = p2/ρg + 6.32/2g + 1.5
- 0 = p2/ρg + 6.32/2g + 1.5
- p2/ρg = – 2.02 – 1.5
- p2/ρg = – 3.52
- p2 = – 3.52 x ρg
- p2 = – 3.52 x 1000 x 9.81 N/m2 (g)
- p2 = – 34531.2 N/m2 (gauge pressure)
Hence, the pressure at point 2 is -34531.2 N/m2(gauge pressure)
Absolute pressure at point 2 = 101325 – 34531.2 = 66793.8 N/m2(absolute pressure)
Video Tutorial: We have made an animated simple video so that there will not be any confusion about gauge pressure, absolute pressure, atmospheric pressure
. You can refer to.
Inverted Siphon
When a siphon is used like U-tube or U-pipe, instead of an inverted U tube, it is called an inverted syphon.
- It’s used based on the requirements if liquid to be pass through a road. This inverted siphon installed below the road
- Here, the liquid has completely filled the pipe
- Flow is due to the pressure instead of gravity flow.

What are the Common Materials of Siphon?
The materials of siphon are based on the applications. Let’s see a few material examples of siphon:
- PVC
- UPVC
- CMP
- HDPE
- Aluminium
- Stainless Steel
- Hastelloy
- Brass, and many more.
Automatic Siphon
If you need intermittent flow, it can be done by using floats, valves, level gauges, levers, sensors, etc. based on the applications. However, do you know that it can be done by siphon as well? Yes, it is! There is one type of siphon that can make automatic intermittent actions with very simple arrangements and it is called an automatic siphon or auto-siphon. Let’s try to understand.
No need so many equipment or auxiliaries for automatic intermittent actions, simple pipe and chamber can make siphoning effects for intermittent actions. Siphon is used and no human intervention is required once it’s started. The automatic siphon works the same as a normal siphon; however, it adds automation so that it can start automatically. It is widely used in
- Beer marking shop
- Toilets
What are the Devices that are not True Siphon?
Some devices which are not true siphons are discussed hereunder.
01. Vacuum Coffee Maker
The device has two chambers. Moreover, gravity and vapor pressure play a crucial role in their operation. It works like a siphon but is not truly a siphon. The working is such that the lower chamber consists of water. The heating and cooling of the lower vessel alter the vapor pressure of the water contained in this lower vessel. As a result, this water moves up to the upper chamber, allowing the water to fall back into the lower chamber. Basically, when the water in the lower chamber is heated, the vapor pressure increases such that at one point, it is higher than the standard atmospheric pressure.
As a result, some water starts boiling and converts into vapor. As we know, the density of vapors is 2000 times lesser than the liquid water, so the vapor and water mixture in this lower chamber expands. When the new pressure becomes higher than the atmospheric pressure, it pushes the leftover water up into the upper chamber, where it stays till the pressure difference between the upper and lower chamber is enough to sustain it.
02. Inverted Siphons
Traps, or inverted siphons, are also not categorized as true siphons. Their construction is such that they constitute a U-shaped pipe that traps gas or liquid while enabling the waste materials to pass easily. They are also defined as pipelines that carry wastewater under the depression in a gravity collection system, such as a valley or roadway or under a building. They are often referred to as depressed sewers. The hydraulic line is below the flow line in a true siphon, while in an inverted siphon, the hydraulic gradient line is above the flow line, which is the basic difference between both.
Therefore, we say that inverted siphons are not true siphons generally. The devices are often used in heating systems to avoid thermos-siphoning. Moreover, they are also utilized in oil refineries, irrigation, and gold mining. Similarly, they are also used to prevent sewer gases from entering the buildings.
03. Siphon Pumps
These are devices capable of using an airtight metering chamber at the crest and a system of automatic valves to discharge fluid at a level higher than the source reservoir. The phenomenon doesn’t require outside pumping energy.
04. Venturi Siphon
It is also called eductor and operates on the venturi effect. When a fluid passes through the constricted area of the pipe, the fluid pressure reduces, and this effect is known as the venturi effect. A typical example is a carburetor.
05. Siphonic Roof Drainage
These devices don’t work as true siphons; instead, they operate on gravity-induced vacuum pumping. Their primary function is to increase flow velocity. Water is carried horizontally from several roof drains to a single downpipe. The most prominent advantage of siphonic roof drainage is that they are cost-effective compared to traditional roof drainage in construction.
Uses or Applications of Siphon
What is a siphon used for? This is used in the following cases,
- To transfer water or any liquid from one reservoir to another reservoir separated by barriers or hills or ridges etc
- To empty a liquid tank that doesn’t have any outlet
- To empty a dam that doesn’t have any sluice gates or any other outlets
- To empty a channel that doesn’t have any sluice gates or outlets.
- To transfer sewerage or stormwater under highways or streams, inverted siphons are widely used.
- Sewage or stormwater under highways or any streams can be transferred by inverted syphon.
- A siphon can purify up to a certain level of liquids. It simply takes the clear liquid without disturbing bottom precipitation or suspended solids at the surface.
- Syphon helps the irrigation system to supply control water from cannel to crop areas.
- Municipal waterworks application
- The siphon effect recovers the pump head due to minor losses for the change in elevation.

Advantages of Siphon or Syphon
The advantages of siphon or syphon are as follows:
- The best thing is that it helps to transfer fluids from one place to another which is separated by high walls or high obstructions.
- The cost of the siphon is very cheap comparatively with pumps etc.
- There will not be any external pressure requirements.
- No power requirements.
- No extra energy cost.
- With the help of a siphon, constant flow is possible.
- No maintenance as well as running cost.
- Since its construction is very simple, it can be easily available in the market.
- There are no rotating parts, and no complexity.
Disadvantages of Siphon or Syphon
The disadvantages of siphon or syphon are as follows:
- Transferring liquid in many cases is not fruitful for syphon due to time. It takes a lot of time. Using a pump will save a good time.
- Siphon or syphon is not generally used in industrial plants as its very slow process.
- In the case of the inverted siphon, the head loss is more in the sewer system
- In the case of an inverted siphon, sedimentation or siltation can block the pipe.
There are so many research on siphon or syphon effects. In 2011, Richert and Binder did the experiment and concluded that siphoning doesn’t depend on the molecular cohesion, instead, it depends on gravity and pressure differential.
They have written: ”As the fluid initially primed on the long leg of the siphon rushes down due to gravity, it leaves behind a partial vacuum that allows pressure on the entrance point of the higher container to push fluid up the leg on that side”.
FAQs on Siphon
What is a siphon used for?
This is a very common question why siphon is used although there are pumps etc. Is it really required? Let’s see the reasons:
If two tanks are kept at different elevations and there is a barrier between them, the siphon is the best suitable choice to transfer liquid from the tank at a higher elevation to the tank at a lower elevation, no need for extra energy here to transfer the liquid. This transfer will be continuous and it will continue until the liquid level in the higher elevation tank reaches the outlet.
Is it siphon or syphon?
Siphon is an Ancient Greek word. It is written as ‘’σίφων’’. In Roman, it is written as ‘síphōn‘, which means “pipe or tube“. It is also spelled as Syphon. Hence, both are correct, however, normally ‘siphon’ is used in maximum cases.
What is siphon in irrigation?
Siphon tubes or pipes are used when two tanks are kept at different elevations and there is a barrier between them. In an irrigation system, there are many instances where barrier comes and water need to transfer from higher elevation to lower elevation.
As siphon doesn’t require any extra energy, it is widely used to transfer liquid wherever possible.
For example: There is a canal and it has water at a higher elevation and land needs to have water which is at a lower elevation. Now, water need to transfer from the canal to your land. You can not cut the canal, right! So, the best option is to use a siphon and transfer water!
Conclusion
So, we have learned syphon along with various working principles, siphon definition, examples, etc. Any questions? Happy learning.


An interesting discussion is value comment. I think that it is best to write more on this topic, it might not be a taboo subject but generally persons are not sufficient to speak on such topics. To the next. Cheers
Hello! I just would like to give a huge thumbs up for the great info you have here on this post. I will be coming back to your blog for more soon.
There is visibly a bundle to know about this. I consider you made certain nice points in features also.
We are a group of volunteers and starting a new scheme in our community. Your site offered us with valuable information to work on. You’ve done an impressive job and our entire community will be grateful to you.